Qubits: The Building Blocks of Quantum Computing

What are Qubits?

Qubits, or quantum bits, are the fundamental units of information in quantum computing. Unlike classical bits, which can only exist in one of two states (0 or 1), qubits can exist in multiple states simultaneously, a phenomenon known as superposition. This unique property allows quantum computers to perform certain computations exponentially faster than classical computers.

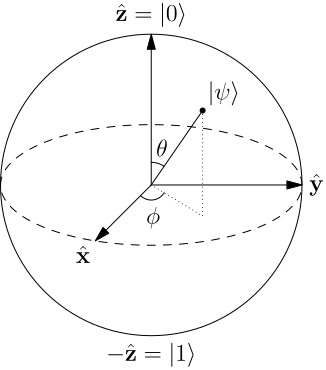
Bloch sphere representation of a qubit. A qubit can be represented as a point on the surface of a sphere, with the north and south poles corresponding to the classical states 0 and 1. (Image: Wikimedia Commons, CC-SA 3.0)

Superposition and Entanglement

Two key properties of qubits that enable quantum computing are superposition and entanglement:

Superposition: Qubits can exist in a linear combination of the states 0 and 1 simultaneously. This allows a quantum computer to perform multiple computations in parallel, leading to a significant speed-up compared to classical computers.
Entanglement: Qubits can become entangled, meaning that the state of one qubit is correlated with the state of another qubit, even if they are physically separated. Entanglement allows for the creation of complex, multi-qubit quantum states and is a crucial resource for quantum algorithms.

Physical Implementations of Qubits

Qubits can be realized using various physical systems, each with its own advantages and challenges:

Superconducting Qubits: These qubits are based on superconducting circuits and are the most widely used in current quantum computers. They are fabricated using lithographic techniques and can be controlled using microwave pulses.
Trapped Ion Qubits: Qubits can be encoded in the internal states of trapped ions, which are held in place using electromagnetic fields. Trapped ion qubits have long coherence times and high-fidelity operations, making them promising for scalable quantum computing.
Photonic Qubits: Qubits can be encoded in the polarization, frequency, or spatial modes of single photons. Photonic qubits are ideal for quantum communication and networking, as they can be easily transmitted over long distances using optical fibers.
Spin Qubits: These qubits are based on the spin states of electrons or nuclei in semiconductors or diamond. Spin qubits can be controlled using magnetic fields and have the potential for integration with classical electronic devices.

Quantum Error Correction

One of the main challenges in building large-scale quantum computers is the fragility of qubits. Qubits are susceptible to errors caused by environmental noise and imperfect control, which can lead to the loss of quantum information. To overcome this challenge, researchers are developing quantum error correction schemes that use multiple physical qubits to encode a single logical qubit, allowing for the detection and correction of errors.

Applications of Quantum Computing

Quantum computers, powered by qubits, have the potential to revolutionize various fields:

Cryptography

Quantum computers can be used to factor large numbers efficiently, which has implications for breaking certain encryption schemes, such as RSA. On the other hand, quantum key distribution protocols, based on the principles of quantum mechanics, can provide provably secure communication.

Drug Discovery and Materials Science

Quantum computers can simulate complex molecular systems, aiding in the discovery of new drugs and materials. By efficiently exploring vast chemical spaces, quantum computers can accelerate the development of personalized medicine and high-performance materials.

Optimization and Machine Learning

Quantum algorithms, such as the Harrow-Hassidim-Lloyd (HHL) algorithm, can solve certain linear systems of equations exponentially faster than classical algorithms. This has applications in optimization problems and machine learning tasks, such as training neural networks and analyzing large datasets.

Challenges and Future Perspectives

Despite the rapid progress in quantum computing, there are still significant challenges to overcome before practical, large-scale quantum computers can be realized. These challenges include improving the fidelity and coherence time of qubits, developing efficient quantum error correction schemes, and scaling up quantum systems while maintaining their performance.

As research in quantum computing continues to advance, we can expect to see the development of more powerful and reliable quantum computers, capable of tackling increasingly complex problems. The integration of quantum computing with classical computing, known as hybrid quantum-classical computing, will also be a key area of investigation, enabling the leveraging of the strengths of both paradigms.