| Posted: Mar 14, 2017 | |
Guiding light with geometric phase |
|
| (Nanowerk Spotlight) The simplest way to trap light, or in general electromagnetic waves, in a finite region is to realize a region surrounded by mirrors so that light is not able to escape. | |
| The simplest mirrors are usually composed of metals. In fact, metallic waveguides are routinely used to guide electromagnetic radiation at low frequency, such as microwaves, for example in the cables carrying the signal from our antenna to the TV. | |
| Nonetheless, in the optical range, metallic waveguides are not useful on long distances owing to the stronger losses, the latter being associated with currents induced in the metal by the electromagnetic wave, than for longer wavelengths. | |
| Long-haul transport of light is at the basis of all the modern communication networks and is based upon transparent materials, technically called dielectrics. | |
| All the known dielectric waveguides are based on the transverse modulation of the refractive index, that is, on the point by point control of the light velocity. The most famous example is the optical fiber, realized in a well-known material, glass. Optical fibers are part of a larger class of waveguides, all of them based on the total internal reflection. | |
| Total internal reflection occurs when light while going from a high (slower speed of light) to a low refractive index region (faster speed of light), is bounced back. The interface between the two materials thus works as a mirror, but free of the losses associated with metals. Other types of dielectric waveguides exist, for example, based upon interferential-based multi-layer mirrors. | |
| All the dielectric waveguides up to now require a transverse modulation of the refractive index, that is, a local control on the speed of photons. | |
| Now, researchers from Portugal, Italy, and Finland, demonstrate the possibility to realize a waveguide without any modulation of the refractive index. They have reported their findings in Nature Photonics ("Guiding light via geometric phases"). | |
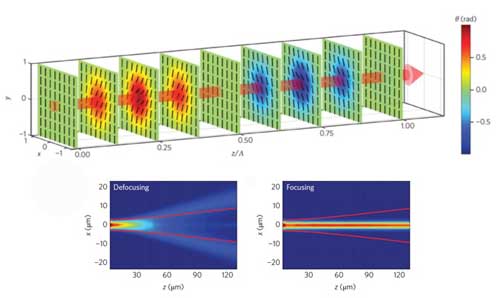 |
|
| Top: sketch of a waveguide based upon the geometric phase. For the sake of simplicity, only some slices of the continuous structure are shown. Black rods represent the local rotation of the material. Confinement is ensured both by a periodic modulation along the propagation distance (axis z) and by a bell shaped distribution in the transverse plane. Bottom: Light propagation in the waveguide, strongly dependent on the input polarization. In fact, for left-handed input polarization light is repelled by the structure, whereas for right-handed polarization transverse confinement occurs. Solid red lines is the beam spreading without the guiding structure. (© Nature Publishing Group) (click on image to enlarge) | |
| The optical delay necessary for the beam confinement is not achieved by a local modulation of the speed of light, but through an exotic effect called geometric phase. | |
| Geometric phase is a temporal delay associated with changes in the propagation of light polarization, the latter corresponding roughly to the oscillation direction of the electromagnetic field. The name geometric is due to the fact that such phase depends exclusively on the geometry of the system, not on the refractive index of the material. | |
| Specifically, geometric phase, in this case, depends only on the rotation of a specific class of materials, called anisotropic media. Given that the local optical delay depends on the point-wise rotation of the material, the transverse phase modulation required to guide light can be realized in an anisotropic material subject to a proper local rotation. | |
| Although the performance of this new type of waveguides is poor with respect to standard fiber optics, the proposed mechanism paves the way for a whole new family of integrated photonic devices, where short range optical propagation is required. | |
| The novel principle for waveguiding, in fact, works at any wavelength, ranging from radio waves to x-rays. It also requires no local change in the refractive index of materials, a requirement hard to satisfy in several materials. These new waveguides support spatially complex modes with respect to standard solutions, making easily accessible an additional degree of freedom for the transport of information on light channels. | |
| By Jisha Chandroth Pannian, University of Porto ([email protected]) and Alessandro Alberucci, Tampere University of Technology ([email protected]) | |
|
Become a Spotlight guest author! Join our large and growing group of guest contributors. Have you just published a scientific paper or have other exciting developments to share with the nanotechnology community? Here is how to publish on nanowerk.com. |
|
