| Posted: January 25, 2008 |
Quasi-crystals avoid repetition |
|
(Nanowerk News) In a crystal, the same atom or group of atoms is repeated with perfect periodicity. Quasi-crystals, on the other hand, are materials without periodicity. Whether the absence of periodicity in a quasi-crystalline material fundamentally affects its properties, however, is still an open question.
|
|
Now, as reported recently in Nature Materials ("Lattice dynamics of the Zn–Mg–Sc icosahedral quasicrystal and its Zn–Sc periodic 1/1 approximant"), an international team of scientists, including Alfred Baron of RIKEN’s SPring-8 Center in Harima, has combined high-resolution neutron and x-ray scattering experiments with theoretical calculations to better understand the physics of quasi-crystals.
|
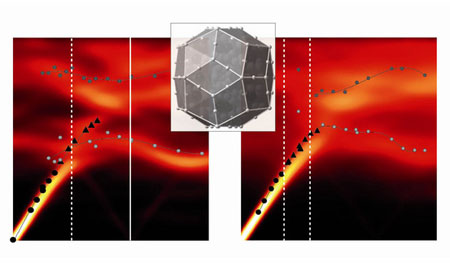 |
| The ‘triacontahedral’ cluster (center) that makes up the i-ZnMgSc quasi-crystal and a crystalline structure—called an approximant—that is chemically similar. The two adjacent panels are a map of the atomic vibrations in the approximant (left) and the quasi-crystal (right). The panels compare atomistic simulations of the atomic vibrations (false color) and the experimental measurements (symbols). (Image; Nature Materials)
|
|
Most of the physics of solids rests on a simplifying assumption, known as Bloch’s Theorem, that can only be applied to crystalline materials. This theorem reduces the task of calculating the properties of macroscopic materials with approximately 1021 atoms to calculating the properties of one repeating unit of atoms. Baron explains: “When Bloch’s theorem no longer applies, the problem becomes much more complex. Quasi-crystals are then interesting both intrinsically, and as a first step away from periodic crystalline order.”
|
|
To tackle the question of how the properties of quasi-crystals may differ from perfect crystals, the researchers focused on a material called i-ZnMgSc. This material consists of ‘triacontrahedral’ clusters each containing 158 atoms and arranged on a quasi-periodic network. i-ZnMgSc is of interest because it has a nearly identical counterpart,or ‘approximant’, made up of similar clusters arranged on a periodic lattice. Thus, comparing the two structures allows researchers to isolate the effects of periodicity—or the lack of it—on materials properties.
|
|
Baron and co-workers used specialized techniques to measure atomic vibrations in i-ZnMgSc and its approximant. The atoms in a material vibrate around their equilibrium positions with patterns and frequencies that are intimately related to the material’s atomic structure, and in particular, the periodicity of this structure. With neutrons, and more recently with x-rays, these vibrations can be measured with very high accuracy.
|
|
The team mapped out the energy of the atomic vibrations for the quasi-crystal and the approximant and compared their results with atomic-scale calculations (Fig. 1). Both the calculations and the experiments show important, though subtle, differences between the two materials. In particular, certain vibrations that are well defined in the approximant have a different energy—or do not even appear—in the quasi-crystal. These results could have relevance to the use of quasi-crystals in several fields, including photonic crystals and thermoelectrics.
|

