Diffraction Limit: The Fundamental Barrier in Optical Microscopy
What is the Diffraction Limit?
The diffraction limit is a fundamental barrier in optical microscopy that sets the minimum size of features that can be resolved using conventional light microscopes. It arises due to the wave nature of light and the physical limitations of focusing light through a lens system. The diffraction limit restricts the spatial resolution of optical microscopes, making it challenging to observe structures smaller than approximately half the wavelength of the illuminating light.
The Physics Behind the Diffraction Limit
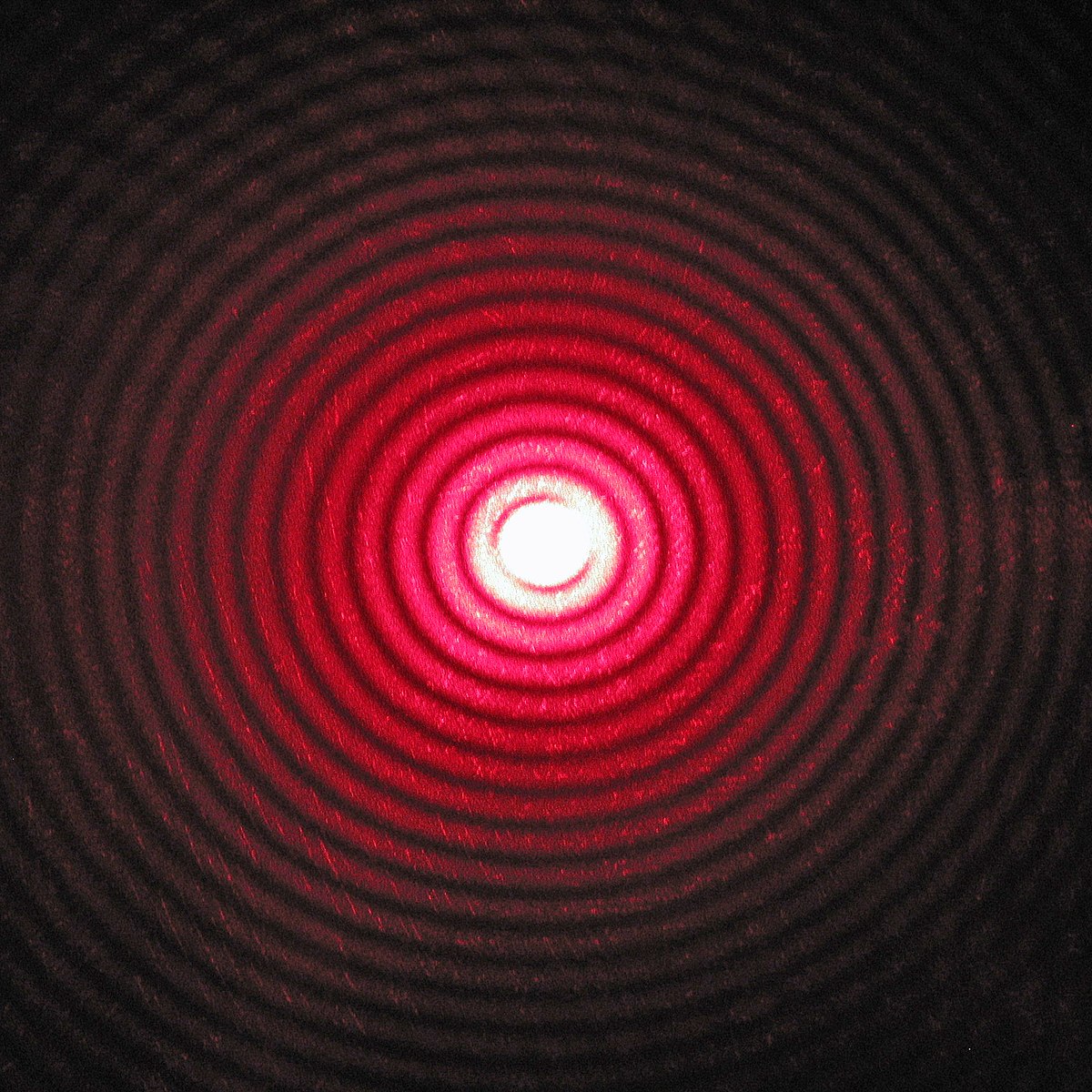
The diffraction limit arises from the wave nature of light and the principles of diffraction. When light passes through an aperture, such as the objective lens of a microscope, it undergoes diffraction and spreads out. This diffraction causes the light to form a diffraction pattern, known as the Airy disk, at the focal plane of the microscope.
Airy Disk and Spatial Resolution
The Airy disk consists of a central bright spot surrounded by concentric rings of decreasing intensity. The size of the Airy disk depends on the wavelength of the light (λ) and the numerical aperture (NA) of the objective lens. The spatial resolution of the microscope is determined by the ability to distinguish two closely spaced Airy disks.
Rayleigh Criterion
The Rayleigh criterion is a commonly used criterion to define the resolution limit of an optical system. According to this criterion, two point sources of light can be resolved when the central maximum of one Airy disk coincides with the first minimum of the other Airy disk. Mathematically, the Rayleigh criterion can be expressed as:
d = 0.61λ / NA
where d is the minimum resolvable distance, λ is the wavelength of the light, and NA is the numerical aperture of the objective lens.
Implications of the Diffraction Limit
The diffraction limit imposes several limitations on optical microscopy:
- Spatial Resolution: Conventional optical microscopes cannot resolve structures smaller than approximately half the wavelength of the illuminating light. For visible light (400-700 nm), this translates to a resolution limit of around 200-350 nm.
- Structural Details: Fine structural details of biological specimens, such as proteins, viruses, and subcellular organelles, are often smaller than the diffraction limit. Consequently, these structures appear blurred or unresolved in conventional optical microscopy.
- Fluorescence Imaging: The diffraction limit also affects fluorescence microscopy, a widely used technique in biological research. Fluorescent probes that are closer than the diffraction limit cannot be resolved as separate entities, limiting the spatial information that can be obtained.
Techniques to Overcome the Diffraction Limit
To overcome the limitations imposed by the diffraction limit, several advanced microscopy techniques have been developed:
Super-Resolution Microscopy
Super-resolution microscopy techniques, such as stimulated emission depletion (STED) microscopy, structured illumination microscopy (SIM), and single-molecule localization microscopy (SMLM), enable imaging beyond the diffraction limit. These techniques employ various strategies, such as patterned illumination, photoswitchable fluorophores, or precise localization of individual fluorescent molecules, to achieve nanoscale resolution.
Near-Field Scanning Optical Microscopy (NSOM)
NSOM is a scanning probe microscopy technique that uses a nanoscale aperture or tip to illuminate the sample in the near-field regime. By collecting the near-field optical signal, NSOM can achieve spatial resolutions well below the diffraction limit, typically in the range of tens of nanometers.
Electron Microscopy
Electron microscopy techniques, such as scanning electron microscopy (SEM) and transmission electron microscopy (TEM), utilize electrons instead of light for imaging. The shorter wavelength of electrons compared to visible light allows for much higher spatial resolution, often reaching the nanometer scale. However, electron microscopy requires specialized sample preparation and is not suitable for live-cell imaging.
Applications and Future Perspectives
Understanding and overcoming the diffraction limit has led to significant advancements in various fields, including:
- Biological Research: Super-resolution microscopy techniques have revolutionized the study of cellular structures, protein interactions, and molecular dynamics at the nanoscale level. These techniques have provided unprecedented insights into the organization and function of biological systems.
- Nanoscience and Nanotechnology: The ability to image and characterize nanoscale structures and devices is crucial for the development of nanotechnology. Techniques that overcome the diffraction limit enable the visualization and manipulation of nanostructures, facilitating the design and optimization of nanomaterials and nanodevices.
- Medical Diagnostics: High-resolution imaging techniques have the potential to improve medical diagnostics by enabling the early detection of diseases at the cellular and molecular level. Super-resolution microscopy can reveal pathological changes in tissues and cells that are not visible with conventional microscopy.
As research continues to push the boundaries of optical imaging, new techniques and technologies are emerging to further overcome the diffraction limit. The development of advanced optics, novel imaging probes, and computational methods will likely lead to even higher spatial resolutions and expanded applications in the future.
